
[Image1]
Introduction
Hey it's a me again @drifter1!
In this article we will continue with Physics, and more specifically the branch of "Classical Mechanics".
Today's article is about Satellite Motion that fits right into the chapter of Gravity.
So, without further ado, let's get straight into it!
Types of Satellites
Satellites are split into two categories: natural satellites and man-made satellites. The moon, planets and coments are natural satellites, whilst man-made satellites are launched into orbit for purposes of telecommunication, scientific research, weather forecasting, intelligence etc. Either way, both types are following the same base physics principles and described by the same math.
Why Satellites don't fall back to Earth?
To understand satellites one has to think of them as projectiles that are only affected by the force of gravity. Once launched into orbit, or while in orbit, the only force that affects their motion is the force of gravity. When the speed of the satellite is sufficient enough then the satellite can orbit around a massive object, like the Earth for example. The motion of the satellite is then circular, or elliptical to be more exact. If the speed is too small it eventually falls back to the Earth. The same also happens if it's velocity is "too parallel" to the gravity force. For the best results the velocity should be perpendicular to the force of gravity.
So, to summarize, its possible to launch a man-made object into space, and hitting the correct criteria of velocity, the then called satellite would start orbiting the Earth, without falling.
The Circular Motion of Satellites
The motion of a satellite can be described as circular as:
- The velocity of the satellite is tangent to the circular path it moves along (and perpendicular to the force of gravity)
- The force of gravity is directed inwards, towards the center of the circular path (or body it orbits)
- The acceleration caused by the force of gravity also points in the same direction
The Relationship of Radius and Velocity
From the physics of circular motion the perpedincular to the motion centripetal forcec is given by the equation:
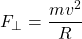
- F⊥: centripetal force
- m: mass of the satellite
- v: velocity of the circular motion
- R: radius of the circular motion

- Fgrav: force of gravity
- G: Gravitational Constant (6.674 × 10-11 N)
- m: mass of the satellite
- M: mass of the central body
- R: distance between the two bodies
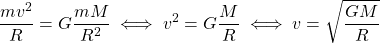
This final equation shows us that the velocity and radius of the satellite's motion are related to each other:
- The closer (R ↓) a satellite is to the central body, the faster it moves (velocity ↑)
- The further away (R ↑) a satellite is to the central body, the slower it moves (velocity ↓)
Acceleration of the Satellite
In previous articles, the acceleration of gravity was proved to be:
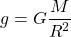
This is the acceleration of the circular motion of the satellite.
Orbital Period
Lastly, an circular motion is also defined by a period T.
From circular motion, its known that the period can be calculated using:
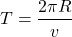
Substituting the equation of velocity v into this equation, :

Its also common to square the whole equation to get:
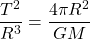
Either way, the period is larger (T ↑) when the radius is larger (R ↑) or the velocity is smaller (v ↓).
Its also worth noting that no equation contains the mass of the satellite, because velocity, acceleration and period are only dependent upon the radius of the orbit and the mass of the central body.
RESOURCES:
References
- https://www.physicsclassroom.com/class/circles/Lesson-4/Circular-Motion-Principles-for-Satellites#:~:text=That%20is%20to%20say%2C%20a,is%20the%20force%20of%20gravity.&text=This%20would%20cause%20the%20projectile,(such%20as%20path%20C)
- https://www.physicsclassroom.com/class/circles/Lesson-4/Mathematics-of-Satellite-Motion
- http://tornado.sfsu.edu/geosciences/classes/m415_715/monteverdi/labs/InclassLab2/u6l4c.html
- http://hyperphysics.phy-astr.gsu.edu/hbase/orbv.html
Images
Mathematical equations used in this article, where made using quicklatex.
Previous articles of the series
Rectlinear motion
- Velocity and acceleration in a rectlinear motion -> velocity, acceleration and averages of those
- Rectlinear motion with constant acceleration and free falling -> const acceleration motion and free fall
- Rectlinear motion with variable acceleration and velocity relativity -> integrations to calculate pos and velocity, relative velocity
- Rectlinear motion exercises -> examples and tasks in rectlinear motion
Plane motion
- Position, velocity and acceleration vectors in a plane motion -> position, velocity and acceleration in plane motion
- Projectile motion as a plane motion -> missile/bullet motion as a plane motion
- Smooth Circular motion -> smooth circular motion theory
- Plane motion exercises -> examples and tasks in plane motions
Newton's laws and Applications
- Force and Newton's first law -> force, 1st law
- Mass and Newton's second law -> mass, 2nd law
- Newton's 3rd law and mass vs weight -> mass vs weight, 3rd law, friction
- Applying Newton's Laws -> free-body diagram, point equilibrium and 2nd law applications
- Contact forces and friction -> contact force, friction
- Dynamics of Circular motion -> circular motion dynamics, applications
- Object equilibrium and 2nd law application examples -> examples of object equilibrium and 2nd law applications
- Contact force and friction examples -> exercises in force and friction
- Circular dynamic and vertical circle motion examples -> exercises in circular dynamics
- Advanced Newton law examples -> advanced (more difficult) exercises
Work and Energy
- Work and Kinetic Energy -> Definition of Work, Work by a constant and variable Force, Work and Kinetic Energy, Power, Exercises
- Conservative and Non-Conservative Forces -> Conservation of Energy, Conservative and Non-Conservative Forces and Fields, Calculations and Exercises
- Potential and Mechanical Energy -> Gravitational and Elastic Potential Energy, Conservation of Mechanical Energy, Problem Solving Strategy & Tips
- Force and Potential Energy -> Force as Energy Derivative (1-dim) and Gradient (3-dim)
- Potential Energy Diagrams -> Energy Diagram Interpretation, Steps and Example
- Internal Energy and Work -> Internal Energy, Internal Work
Momentum and Impulse
- Conservation of Momentum -> Momentum, Conservation of Momentum
- Elastic and Inelastic Collisions -> Collision, Elastic Collision, Inelastic Collision
- Collision Examples -> Various Elastic and Inelastic Collision Examples
- Impulse -> Impulse with Example
- Motion of the Center of Mass -> Center of Mass, Motion analysis with examples
- Explaining the Physics behind Rocket Propulsion -> Required Background, Rocket Propulsion Analysis
Angular Motion
- Angular motion basics -> Angular position, velocity and acceleration
- Rotation with constant angular acceleration -> Constant angular acceleration, Example
- Rotational Kinetic Energy & Moment of Inertia -> Rotational kinetic energy, Moment of Inertia
- Parallel Axis Theorem -> Parallel axis theorem with example
- Torque and Angular Acceleration -> Torque, Relation to Angular Acceleration, Example
- Rotation about a moving axis (Rolling motion) -> Fixed and moving axis rotation
- Work and Power in Angular Motion -> Work, Work-Energy Theorem, Power
- Angular Momentum -> Angular Momentum and its conservation
- Explaining the Physics behind Mechanical Gyroscopes -> What they are, History, How they work (Precession, Mathematical Analysis) Difference to Accelerometers
- Exercises around Angular motion -> Angular motion examples
Equilibrium and Elasticity
- Rigid Body Equilibrium -> Equilibrium Conditions of Rigid Bodies, Center of Gravity, Solving Equilibrium Problems
- Force Couple System -> Force Couple System, Example
- Tensile Stress and Strain -> Tensile Stress, Tensile Strain, Young's Modulus, Poisson's Ratio
- Volumetric Stress and Strain -> Volumetric Stress, Volumetric Strain, Bulk's Modulus of Elasticity, Compressibility
- Cross-Sectional Stress and Strain -> Shear Stress, Shear Strain, Shear Modulus
- Elasticity and Plasticity of Common Materials -> Elasticity, Plasticity, Stress-Strain Diagram, Fracture, Common Materials
- Rigid Body Equilibrium Exercises -> Center of Gravity Calculation, Equilibrium Problems
- Exercises on Elasticity and Plasticity -> Young Modulus, Bulk Modulus and Shear Modulus Examples
Gravity
- Newton's Law of Gravitation -> Newton's Law of Gravity, Gravitational Constant G
- Weight: The Force of Gravity -> Weight, Gravitational Acceleration, Gravity on Earth and Planets of the Solar System
- Gravitational Fields -> Gravitational Field Mathematics and Visualization
- Gravitational Potential Energy -> Gravitational Potential Energy, Potential and Escape Velocity
- Exercises around Newtonian Gravity (part 1) -> Examples on the Universal Law of Gravitation
- Exercises around Newtonian Gravity (part2) -> Examples on Gravitational Fields and Potential Energy
Final words | Next up
And this is actually it for today's post!
Next time we will get into Kepler's Laws of Planetary Motion...
See ya!


