[Image 1]
Introduction
Hey it's a me again @drifter1! Today we continue with Physics and more specifically the branch "Classical Mechanics" to talk about Internal Energy and Work. So, without further ado, let's get straight into them!
Internal Energy
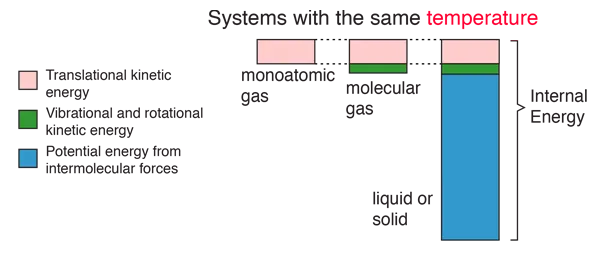
Talking about the Physics branch which is called "Thermodynamics", Internal energy is defined as the property or state that a specific substance has on absence of capillarity (the tendency of a liquid to rise or fall as a result of surface tension) and external electric, magnetic or other types of fields. That way, according to the first law of thermodynamics, any change that a system undergoes is the result of some work, with this work being equal to the change in internal energy. Sometimes this internal energy can be represented as the sum of kinetic, potential and chemical energy. That's exactly why I wanted to cover this topic :)Let's now think about a glass of water that's standing still on top of a table. Let's suppose that we don't move this glass, which means that there will be no change in kinetic energy (KE = 0) and that potential energy can be considered zero (PE = 0). Does this glass have any energy now? Yes, it has chemical energy, which happens in the microscopic scale and is caused by the random, disordered motion of the water's molecules. So, there's surely no apparent energy in the macroscopic scale, but thinking about the microscopic scale we understand that there's some microscopic kinetic energy caused by the motion of the molecules and also some microscopic potential energy caused by the attractive and repetitive forces between the water molecules.

[Image 2]
Internal Work
Talking about any physics system there will be forces that are exerted by the surroundings (external) and also forces which are part of the same system (internal). For these two kinds of forces we define the corresponding terms of external work and internal work. This categorization is very important as it helps us understand which forces are conservative and which not (non-conservative).Simplifying the whole topic we can say that external forces include the applied force, normal force, tension force, friction force and air resistance force. In the same notion, internal forces include the gravity force, magnetic force, electric force and spring force, which as you might remember from previous articles are all considered conservative forces. Talking about internal forces, we can apply the so called conservation of energy theorem, which tells us that the total (mechanical) energy of a system gets conserved over time.
RESOURCES:
References
- https://www.britannica.com/science/internal-energy
- http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/inteng.html
- https://physics.info/work/
- https://www.physicsclassroom.com/class/energy/Lesson-2/Internal-vs-External-Forces
Images
- http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/inteng.html
Mathematical equations used in this article, where made using quicklatex.
Previous articles of the series
Rectlinear motion
- Velocity and acceleration in a rectlinear motion -> velocity, accelaration and averages of those
- Rectlinear motion with constant accelaration and free falling -> const accelaration motion and free fall
- Rectlinear motion with variable acceleration and velocity relativity -> integrations to calculate pos and velocity, relative velocity
- Rectlinear motion exercises -> examples and tasks in rectlinear motion
Plane motion
- Position, velocity and acceleration vectors in a plane motion -> position, velocity and accelaration in plane motion
- Projectile motion as a plane motion -> missile/bullet motion as a plane motion
- Smooth Circular motion -> smooth circular motion theory
- Plane motion exercises -> examples and tasks in plane motions
Newton's laws and Applications
- Force and Newton's first law -> force, 1st law
- Mass and Newton's second law -> mass, 2nd law
- Newton's 3rd law and mass vs weight -> mass vs weight, 3rd law, friction
- Applying Newton's Laws -> free-body diagram, point equilibrium and 2nd law applications
- Contact forces and friction -> contact force, friction
- Dynamics of Circular motion -> circular motion dynamics, applications
- Object equilibrium and 2nd law application examples -> examples of object equilibrium and 2nd law applications
- Contact force and friction examples -> exercises in force and friction
- Circular dynamic and vertical circle motion examples -> exercises in circular dynamics
- Advanced Newton law examples -> advanced (more difficult) exercises
Work and Energy
- Work and Kinetic Energy -> Definition of Work, Work by a constant and variable Force, Work and Kinetic Energy, Power, Exercises
- Conservative and Non-Conservative Forces -> Conservation of Energy, Conservative and Non-Conservative Forces and Fields, Calculations and Exercises
- Potential and Mechanical Energy -> Gravitational and Elastic Potential Energy, Conservation of Mechanical Energy, Problem Solving Strategy & Tips
- Force and Potential Energy -> Force as Energy Derivative (1-dim) and Gradient (3-dim)
- Potential Energy Diagrams -> Energy Diagram Interpretation, Steps and Example
Final words | Next up
This is actually it for today's post! I'm not quite sure about next time. It's possible that we will get into the next topic of momentum, but maybe I will first get into exercises around the whole conservation of energy stuff :)
See ya!


Keep on drifting!
